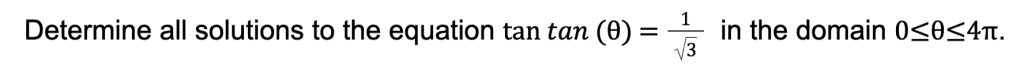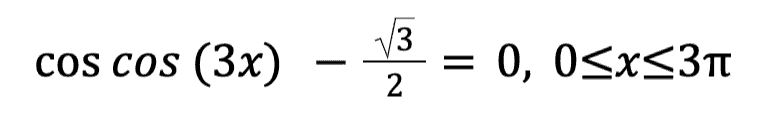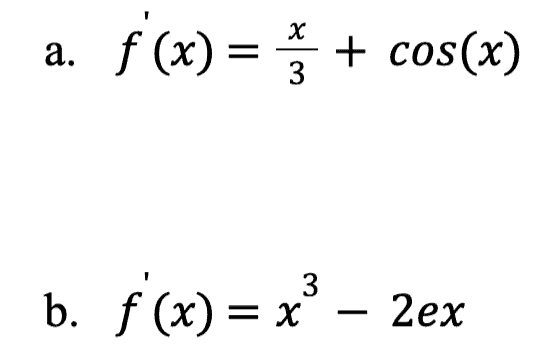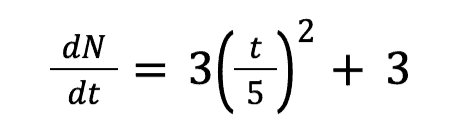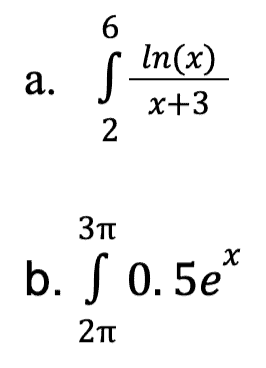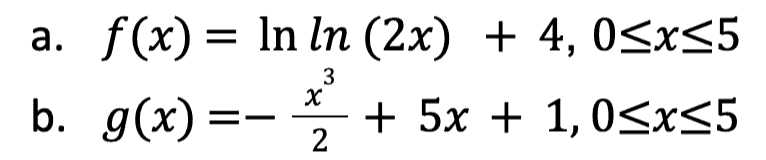QCAA Practice Questions for Unit 3 & 4 Maths Methods External Assessment

Question 6 (2 marks)

a. If f(x) = -ln ln(x) + 2x² determine f'(x)

b. Using your answer for f'(x) from part (a), determine f”(x)

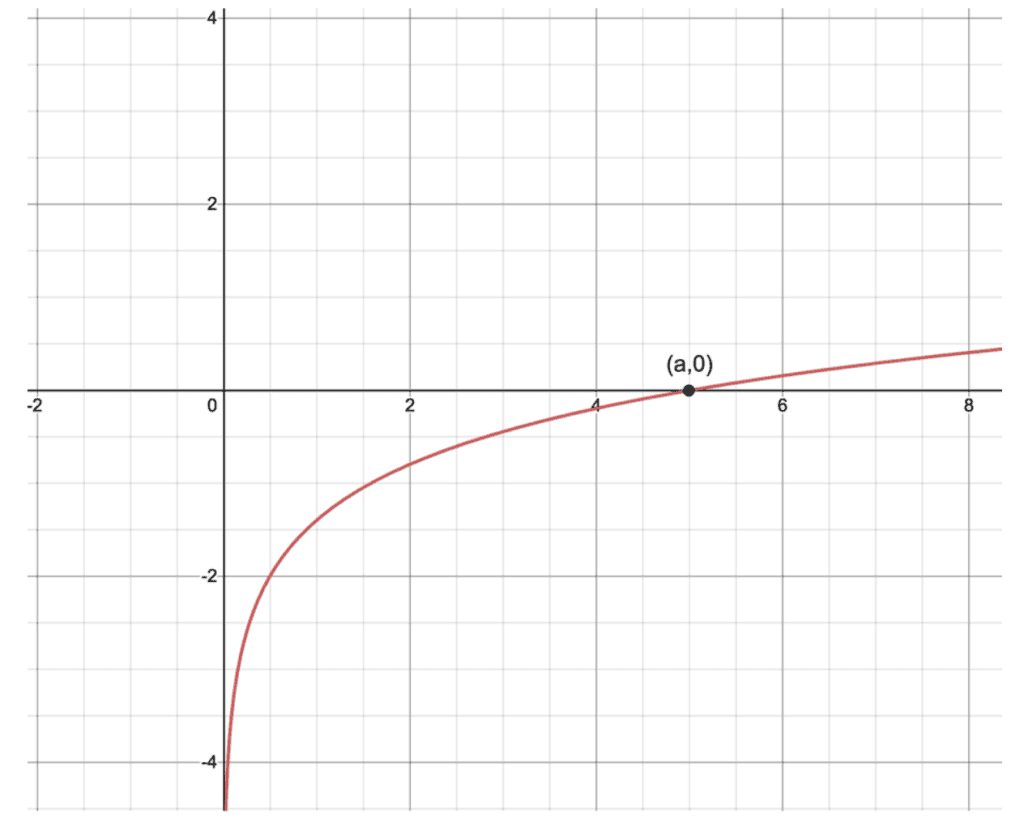
Question 7 (4 marks)

The graph of the function f(x) = 2log(2x) – 2 is shown below. 

a. Determine the value of a given that it is the x-intercept.

b. State the domain and range of the function.

c. Determine the gradient of the curve at (a,0). Give your answer to two decimal places.

d. Determine the equation of the tangent at (a,0). Give your answer to two decimal places.

Question 8 (4 marks)

The depth (d) of a water in 100m from the shore of a beach changes with the tide according to the following rule:

where t is the time in hours after high tide and depth is measured in meters. If on a particular day, the high tide is at 7:00 pm:

a. By sketching the curve, calculate the depth of the water at low tide and when it will next occur

b. Determine the rate of change of the depth of water with respect to time at 10:00 pm

Question 9 (3 marks)

Given that f(x) = 3x(x + 5)², evaluate f'(x) and determine the equation of the tangent to the curve at point (0,0)

Question 10 (4 marks)

The temperature (T) in Sydney in °C on a summer day can be modelled by the following function:

T = 5sin sin (t – 3) + 25, 0 ≤ x ≤ 24

where t is the number of hours after 12am (start of that day)

a. Calculate the temperature at 7am. Give your answer to the nearest degree.

b. Calculate the rate of change at any time

c. What is the rate of change of temperature at 6pm? Give your answer correct to two decimal places.

Question 11 (2 marks)

Determine f(x) of the following 

Question 12 (3 marks)

The population of rabbits in a field changes at a rate modelled by the following function:

where:

• N is the number of rabbits in the field 
• t is the number of days after the first rabbits arrived at the field 

a. Determine the rule for the population of tadpoles at a given time if initially there were 3 rabbits

b. What is the rabbit population after 6 days? Round answer to the appropriate whole number

Calculator Allowed Questions

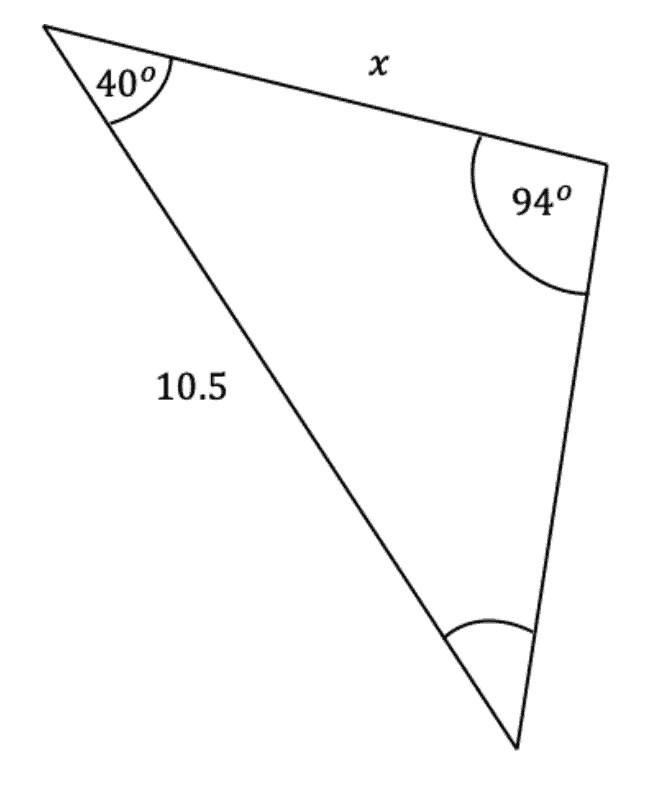
Question 13 (2 marks)

Find the length of the side labelled x. Give your answer to two decimal places.

Image sourced from MathsMadeEasy

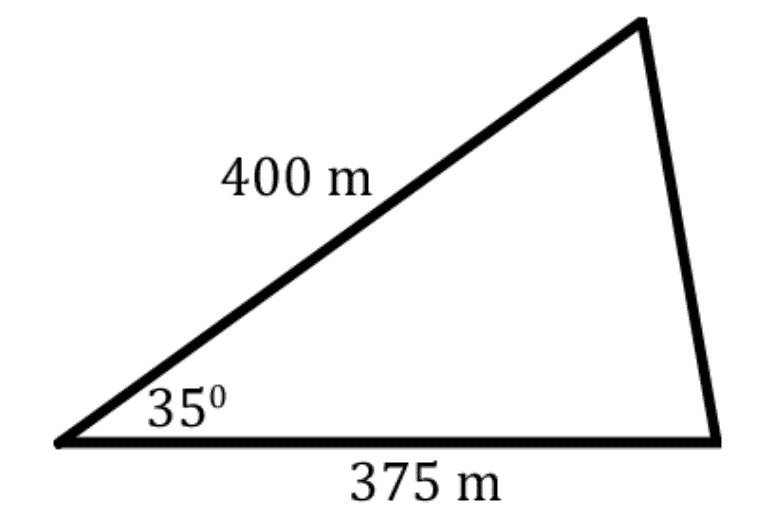
Question 14 (2 marks)

Find the length of the unknown side. Give your answer to two decimal places.

Image sourced from MATHGuide

Question 15 (3 marks)

Jason bought a weighted dice. After rolling the dice 50 times, he found that it landed on 6 40% of the time. Letting X be the dice landing on 6:

a. Construct a probability distribution table for X

b. Determine E(X)

c. Determine the variance and standard deviation of X, correct to four decimal places

Question 16 (5 marks)

Sketch the graph of f(x) = 2ex³ + 2x + 3. Calculate and label the x and/or y-intercepts, stationary points and points of inflection. Give your answers to two decimal places. 

Question 17 (2 marks)

Z is a normally distributed random variable, determine:

a. x given that P(Z > x) = 0.73, Z ~ N (7.4, 1.1²)

b. n given that P(Z < n) = 0.34, Z ~ N (21, 5.3²)

Question 18 (4 marks)

Kate surveys 175 people and finds that 95 of them take public transport to work. Estimate the proportion of the population that does not take public transport to work. Determine a 98% confidence interval. Give your answers to four decimal places. 

Question 19 (2 marks)

On average, 40% of people spend at least 200 hours a year driving. Using the normal approximation, determine the approximate probability that, in a sample size of 600, more than 60% of people have spent at least 200 hours a year driving. 

Question 20 (3 marks)

A deposit of $7,500 is invested at Bank A, and a deposit of $10,000 is invested at Bank B. Bank A offers compound interest continuously at a rate of 7% per annum, whereas Bank B offers compound interest continuously at a rate of 4% per annum. In how many years will the two investments be the same? Give your answer to the nearest appropriate year. Use the compound interest equation below: 

A = Pe^rt

where:

• A = accumulated dollars 
• P = initial deposit 
• r = rate 
• t = years 

Question 21 (2 marks)

Solve the following. Give your answer to two decimal places. 

Question 22 (1 mark)

The area of the region enclosed by the graphs of y = -(2x – 2)² + 8 and y = x³ + 4x + 3 to two decimal places is…

Question 23 (3 marks)

Calculate the values of x for which the following functions have the same gradient

Question 24 (5 marks)

Consider the function f(x) = ln ln (2x) + 3

a. Determine the coordinates of the x-intercept(s) and y-intercept(s)

b. Sketch and label the function with all important features

c. State the transformations required to map f(x) = ln⁡(x) onto f(x) = ln ln (2x) + 3

Question 25 (2 marks)

Solutions to Maths Methods EA Practice Questions

Click here to download the solutions to the 25 Maths Methods EA practice questions above!